Discrete Review
Answer Sheet
Writing Assignment
3d
\[\{ (x, y): x = y \}\]reflexive, symmetric, antisymmetric, transitive
- reflexive: $x = x$
- symmetric: $x = y \implies y = x$
- antisymmetric: $x = y \land y = x \implies x = y$
- transitive: $x = y \land y = z \implies x = z$

16a
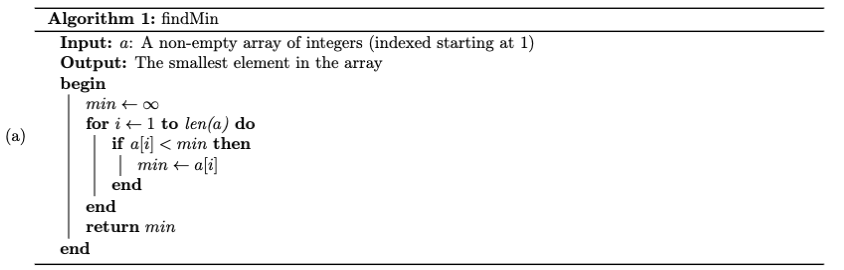
Invariant
At the end of step i of the loop, min always contains the smallest element of the array from index 1 to i of a.
- Initialization: In the first iteration, min = a[1], which is the smallest element of the array from index 1 to 1 of a.
- Induction: Assume that the invariant holds at the end of step k of the loop (min is the minimum value of a[1, …, k]). At the k+1 step, min is the minimum value of a[1, …, k] and a[k+1]. So, min is the minimum value of a[1, …, k+1].
Soundness
When termination occurs, i = len(a). Since the invariant holds, min is the minimum value of a[1, …, len(a)]. So, min is the minimum value of a.
Correctness
For each iteration, i is incremented by 1 and getting close to the end of a. When i = len(a), the loop terminates.
16b
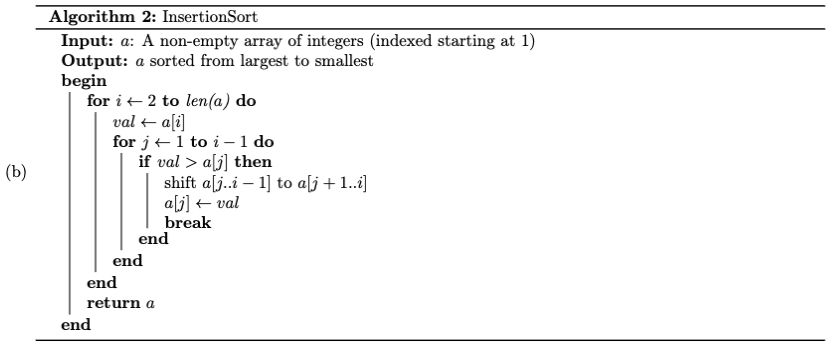
Invariant
At the end of each outer loop, the array a[1, …, i] is sorted.
- Initialization: In the first iteration, a[1] is sorted.
- Induction: Assume that the invariant holds at the end of the k-th iteration of outer loop (a[1, …, k] is sorted). In k+1-th iteration of outer loop, at the beginning of the inner loop, a[1, …, k] is sorted. At the end of the inner loop, a[k+1] is inserted into the correct position so that a[1, …, k+1] is sorted. So, a[1, …, k+1] is sorted at the end of the k+1-th iteration of outer loop.
Soundness
When termination occurs, i = len(a). Since the invariant holds, a[1, …, len(a)] is sorted. So, a is sorted.
Correctness
For outer loop, i is incremented by 1 and getting close to the end of a. When i = len(a), the loop terminates.
For inner loop, j is decremented by 1 and getting close to the beginning of i-1. When j = i-1, the loop terminates.